Best Difference Between Coefficient and Constant

Coefficient and Constant: There are two possible outcomes when two things change simultaneously, the first of which being that their values change simultaneously; for instance, children grow taller as their age does - their height increases with each year they live through. Second, two things may move in opposite directions. A higher magnitude results in greater value being assigned for another property (vice versa); both properties being negatively related. When an object's value remains constant over time it's considered constant while its variable properties indicate variable relationships based on conditions or situations (variable properties or coefficients). This article attempts to outline all differences between constants and coefficients by providing examples and scenarios of each pair to help.

What is The meaning of Coefficient?

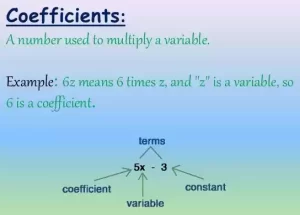
A coefficient in mathematics refers to any number or algebraic factor multiplied by one or more variables within an algebraic expression or equation. It measures scale or proportionality among variables found within an expression and allows researchers to accurately determine each variable's contribution by multiplying by its coefficients.

Calculus, algebra, and linear equations all rely heavily on coefficients as crucial tools for data gathering about variables relationships; and allow operations on expressions to take place mathematically. The use of coefficients has become pervasive across these disciplines of math as their purposeful use allows an expression's values to be easily computed with multiple computations simultaneously.

In an expression such as 3x +2y - 5, for instance, two coefficients stand out, coefficient 2 multiplies variable by three while 3 multiplies it by five; these respective multipliers determine which side has greater weight based on how their respective coefficients interact with variables x and y respectively.

Coefficients are integers that may be positive or negative and come in various forms such as fractions, decimals and algebraic expressions. Their values determine how equations and expressions behave with those which contain them.

What is The meaning of Constant?

A constant is defined in mathematics as any value which does not change over time - fixed values that cannot change.

Constants can be used in formulas and equations to represent quantities or parameters without depending on variables; their values remain fixed over time, helping define mathematical relationships more precisely.

Constants can be classified into two main types

Numerical constants are values used in mathematical formulae.

Examples may include:

• Numerical Constants: Integral constants consist of whole numbers such as 3, 7, or 20 for example.
• Decimal constants: Decimal constants are fractional numbers such as 2.5 or 0.75.
• Pi (π): Pi (p), the mathematical constant representing the ratio between a circle's diameter and circumference, stands at approximately 3.14159.
• Euler's Number (e): this is the base of the natural logarithm, approximately equaling 2.7182.
• The golden ratio (φ): Golden Ratio (ph), is a mathematical constant with numerous geometric and algebraic properties that approximates 1.61800 in value.
• Constants Symbolic: Constants represented as letters or symbols can represent specific values; symbolic constants can often be found within algebraic expressions such as these

examples:

• Physics defines light's speed as 299.792,458 metres per second - this symbolism of its acceleration being represented by "c".
• Gravitational acceleration can be represented as "g", with 9.8 meters/second squared being its equivalent value.

Constants are vital because they offer fixed values and information that help solve mathematical issues mathematically, generate formulae and establish relationships among variables. Constants play an essential role in various branches of mathematics, engineering, physics and other sciences.

Importance of understanding the difference between coefficient and constant

Understanding the difference between constants and coefficients is integral in math and algebra, for many reasons:

1. Proper Expression Evaluation: Constants and coefficients must be differentiated when analyzing algebraic equations to achieve accurate calculations with reliable results. Constants represent fixed values while coefficients show scale or proportionality - an accurate calculation requires understanding which parts represent constants vs coefficients in your formulae.
2. Algebraic Manipulation: Manipulation of algebraic expressions includes operations such as factoring, simplification, and expansion. Knowledge of constants and coefficients will allow you to recognize terms with similar properties that can be combined together for easier algebraic manipulations and expression simplification. This knowledge gives rise to effective manipulations as well as simplification.
3. Solving Equations: Solving Equations Equations include variables, coefficients and constants which you can solve by identifying their roles within an equation. Locating coefficients will assist with locating unknowns; while constants remain fixed throughout this process of solving equations.
4. Interpretation of Real:  In real-life scenarios, algebraic equations or expressions often represent complex situations accurately. You can interpret their meaning by differentiating constants from coefficients; for instance, in an equation representing cost functions the constants might represent fixed costs while their coefficient could represent item price differences.
5. Communication and Collaboration: Being familiar with constants and coefficients will facilitate clear, unambiguous discussion when solving math-based problems, as well as accurate interpretation and calculations among teams or individuals. It ensures effective dialogue about mathematics that allows teams or individuals to work more collaboratively together on solving those same issues.
6. Beyond Constants and Coefficients: Constants and coefficients provide the basis of more advanced topics like polynomial functions, systems of equations and calculus. Understanding this concept will lead to further success in learning these topics.

Understanding the difference between constants and coefficients is vitally important if you hope to become proficient at expression evaluation, algebraic manipulation, equation solving, real-world problem analysis, and effective communication. An in-depth knowledge of this concept will elevate both mathematical reasoning and problem-solving abilities.

Coefficient

Coefficient In an algebraic equation or expression, a coefficient is an integer or algebraic number which multiplies one variable against itself to represent scale or proportionality within an expression; its values reveal how each variable contributes towards the total expression value.

Here are some key points about coefficients:

1. Definition of Constant Multipliers in Equations or Expressions: A constant multiplier is any multiplier that remains constant between specific variables in an expression or equation.
2. Role in Algebraic Expressions: Coefficients play an integral part in algebraic expressions; they define the magnitude or weight of variables, as well as show their multiples.
3. Example: In the equation 3x + 2y -5 the coefficients total three. Variable x multiplies coefficient three while variable 2 multiplies coefficient two.

Types of Coefficients:

1. Numeric Form: When considering numerical coefficients such as 4x for instance, its coefficient can be represented with four.

2. Algebraic Coefficients: These coefficients may include variables, or they could even be expressions, such as 2xy for instance which has two coefficients in this form, twoxy.

Sign of Coefficients Coefficients may take three forms: positive, zero, or negative. Their sign indicates the magnitude and direction of a relationship between variables and expressions, A positive coefficient indicates a variable has a positive impact, while negatively means it has negative ramifications; zero coefficients show they do not impact expressions at all.

Importance in Equations: Coefficients play an essential role in solving equations; they allow us to isolate variables and define their values accurately, as well as finding solutions by manipulating equations or manipulating coefficients.

Coefficient and Variable Independence: Within an expression, coefficients and variables exist as independent entities that exist as separate elements within it. They each exist on their own within their composition.

Understanding coefficients will allow you to evaluate expressions, manipulate algebraic equations and solve mathematical problems more quickly and accurately. They provide essential insight into the relationships among variables as they impact an expression.

Constant

Constants (constants in French) occupy an unchanging value within any context or problem, whether numerical or symbolic values that do not change over time or vary as part of any given solution or solution set.

Here are some key points about constants:

1. Definition of Constant Values: Constant values refer to values that remain static regardless of circumstances or situations they arise in, remaining constant throughout calculations or mathematical operations.
2. Role in Algebraic Expressions: Constants play an integral part of algebraic expressions. Constants are terms that do not multiply with any variables - they represent known quantities that do not change over time.
3. Example: In 3x + 2y = 5, 5, as an absolute constant is used and does not involve any variables or shifting values.

Types of Constants:

1. a.Numeric Constants - Numeric constants are numbers used for calculations, including decimals such as 3.14 or integers such as 2 or 7.
2. b. Symbolic Constants - These constants can be represented using letters or symbols representing specific values; for instance, when solving for light speed (c), constant "c" stands in for an absolute value.

Invariant Value: Constants are constants with an invariant value; that is, they remain constant no matter the circumstances or problems encountered while using them. They remain true no matter which calculations or operations take place on them or in what context or problem setting.

Application: Constants can be used in formulas and equations to represent quantities or parameters without depending on variables; providing fixed values that make problem solving and mathematical computation simpler.

Interpretation: Constants can have specific mathematical or physical meaning. Examples include the speed of light in physics (c), gravitational coefficient (G) and Planck's Constant (h).

Constants are essential tools in accurately representing and interpreting expressions, equations and formulas. Constants provide known values which enable one to define relationships among variables or evaluate mathematical system behavior as well as solve various scientific and mathematical disciplines' related problems.

Key Differences between Coefficient and Constant

Mathematically speaking, constants and coefficients differ substantially in various respects; here are a few key distinctions:

Definition and Purpose:

1. Coefficient: A coefficient is an algebraic or numeric factor which multiplies a variable within an expression or equation to scale or proportion the variables proportionality or size.
2. Constants: Fixed values refer to quantities which remain constant within any specific environment or situation; for instance, those which don't change through an expression.

Search an Expression:

1. Coefficient: They can also be added directly into expressions by multiplying coefficients with variables.
2. Constants: Constants can be used as standalone terms within an expression and do not need to be multiplied with variables.

Variables and Coefficients:

1. Coefficient: Coefficients can be affected by variables and their values may change accordingly, providing valuable information about the relationship between variables and expressions.
2. Constants: Constants remain consistent regardless of any changes to variables' values; they have fixed representation either numerically or symbolically and do not depend on changes made by variables.

Impact Analysis : 

1. Coefficient: Coefficients are mathematical quantities used to scale variables to define an expression's value and examine their influence over time.
2. Constants: Constants are fixed values used to add or subtract from an expression independently of variables. They provide a fixed, known value which adds stability and predictability to its total amount.

Constants are values which don't depend on variables; on the other hand, coefficients determine scale or proportionality among variables in an expression. Constants, though associated with particular variables, remain independent terms but their value changes with those variables; their contribution remains constant while coefficients influence its impact. Accurate evaluation of algebraic equations and expressions requires understanding both these concepts.

Importance of Recognizing the Difference

Understanding the difference between constants and coefficients is of vital importance; knowing this distinction allows one to make informed decisions when performing calculations with these terms.

It is thus imperative to know their difference:

1. Calculate Your Expression Accurately: By distinguishing constants from coefficients, algebraic expressions can be evaluated accurately using proper mathematical operations to produce appropriate results. Accuracy also comes from accurately identifying constants as constants when performing evaluation calculations.
2. Algebraic Manipulation: Acknowledging the difference between constants and coefficients allows for effective manipulation of algebraic terms, helping simplify equations through combination. Constants should be identified so as to maintain their fixed values while still permitting algebraic manipulation.
3. Solving Equations: Coefficients and constants play vital roles when solving equations. A good grasp of coefficients will enable you to isolate variables, determine their values and speed up the solution process; while constants maintain their values during equation solving and may provide solutions.
4. Interpreting Real-World Problems: Algebraic equations or expressions provide a useful model of many real-world issues, so knowing the difference between constants and coefficients is vital in grasping their impactful significance in regards to problem components. You can apply your knowledge by understanding relationships between fixed quantities and variable ones - an approach to real world applications!
5. Effective Communication and Collaboration: Understanding the difference between constants and coefficients will enable you to communicate and collaborate efficiently in math discussions and problem-solving tasks, providing clear explanations, interpretations and sharing of mathematics concepts, results and solutions.
6. Advanced Mathematical Concepts: Understanding coefficients and constants will enable you to better grasp advanced mathematical topics, including polynomial functions, systems of equations calculus linear algorism among others. By being aware of their differences you can establish a firm foundation for such advanced topics.

Understanding the difference between constants and coefficients is vitally important for accurately evaluating expressions, manipulating algebraic equations effectively, solving problems efficiently, interpreting real world situations appropriately and moving onto more advanced concepts. Gaining this knowledge will allow you to perform mathematical operations correctly while efficiently analyzing problems as well as communicating these ideas effectively.

Conclusion

Understanding the distinction between constants and coefficients in mathematics is vital. Constants represent constant values while coefficients represent scale or proportionality within algebraic expressions; understanding this allows for accurate evaluation and manipulation of algebraic formulas. At its heart is its capability of precise algebraic manipulation and effective equation solving, aiding real world applications of mathematical concepts while providing communication and collaboration during mathematical discussions and task completion.

Learning constants and coefficients provides a solid basis for exploring more advanced mathematical topics like polynomial function, systems of equations calculus, linear algorithms and others. Understanding the difference between constants and coefficients will enable you to express mathematical ideas more clearly, so continue studying these concepts to further your knowledge in mathematics.